Efficiency and Fuel Consumption Costs for Irrigation Pumps
Guide M-227
Blair Stringham
College of Agricultural, Consumer and Environmental Sciences, New Mexico State University
Author: College Associate Professor, Department of Plant and Environmental Sciences, New Mexico State University. (Print friendly PDF)
Origin of the Horsepower Unit
Before steam power became widespread during the Industrial Revolution, horses were the main source of energy for applications like pulling carts, turning grinding mill wheels, and providing movement to industrial machines. As the availability of steam engines increased, a means of providing understandable power ratings became important. Comparing the power output of a steam engine to a corresponding number of horses was an easy way for prospective engine owners to understand and compare power ratings. James Watt, an engineer, inventor, and entrepreneur of the late 1700s, determined that by recording the distance a horse traveled in a specific time while pulling a known weight against gravitational force, a measurement could be made of the power the horse produced. After several observations, Watt concluded that a strong horse could provide 550 foot-pounds per second of power, or one horsepower (Figure 1).
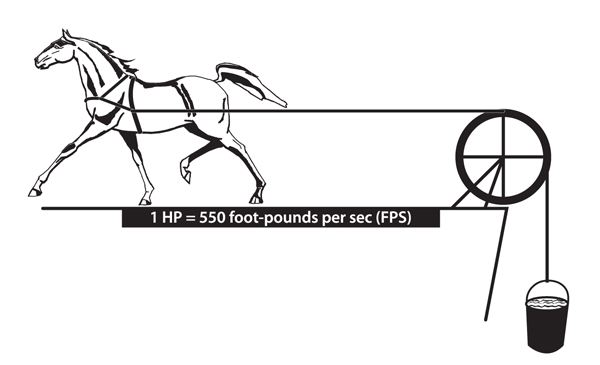
Figure 1. Diagram demonstrating the definition of horsepower.
Water and Pump Horsepower
To fully understand water horsepower, it is important to understand the terminology involved in deriving such a unit. The term "energy" is defined as the capacity to do work. "Power" is the rate at which energy flows or at which energy is used per unit of time; it is also the rate at which work is performed. In other words, power is the amount of energy that is used to do work or how quickly work can be done. Water horsepower is the minimum power that is required to move the water (Figure 2). In other words, it is the power that the pump would require if the pump were 100% efficient. The water horsepower can be determined if the flow rate of the water and the force (pressure) required to produce that flow are known.
The following formula can be used to calculate water horsepower (WHP).
WHP = HQ / 3960
Where H is the change in pressure measured in height of water in feet and Q is the water flow rate in gallons per minute.
This equation was derived knowing that one horsepower is equal to 550 ft-lb/s.
For example, if an irrigation pump located at ground level is pumping 460 gallons of water per minute from a well whose water level is 112 feet below the ground level and discharging that water at ground level, the water horsepower that the pump is delivering is:
WHP = (112 x 460) / 3960 = 13.0
So the pump is providing 13.0 WHP when it lifts water 112 feet at a rate of 460 gallons per minute.
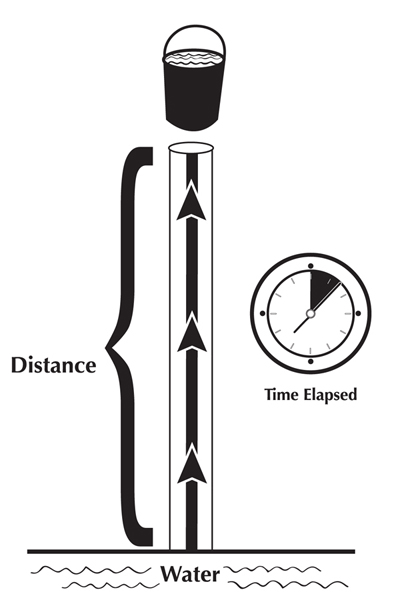
Figure 2. Diagram illustrating water horsepower by moving a volume of water a specified distance per period of time.
Pump Efficiency
No pump can convert all of its mechanical power into water power. Mechanical power is lost in the pumping process due to friction and other physical losses. It is because of these losses that the horsepower going into the pump must be greater than the water horsepower leaving the pump. The efficiency of any given pump (η) is a ratio defined as the water horsepower out divided by the mechanical horsepower into the pump.
η = water hp out / hp into pump 0 < η < 1
If the pump in the last example uses 17.0 HP to provide 13.0 WHP, the pump efficiency is:
η = 13 / 17 = 0.76 or 76%
The pump is 76% efficient, and 24% of the input energy is lost to friction and other losses. Most modern pumps have an efficiency of 50 to 85%.
When choosing a pump, it is important to consider the relationship between efficiency and overall cost. More efficient pumps tend to be more expensive. However, with better efficiency comes lower fuel costs to run the pump. Although more efficient pumps usually come with an increase in capital cost, the overall fuel consumption will be lower, resulting in lower annual fuel or electricity costs.
It should also be noted that the discussion in the previous paragraphs was for a pump properly sized for the application. If the pump does not match the application, it may have to operate in an inefficient range, and fuel or electricity will be wasted. Consult with a Professional Engineer or a pump supplier if you have questions about a specific pump or application.
References
Dickinson, H.W. 1936. James Watt: Craftsman and engineer. Cambridge: Cambridge University Press.
Hart, I. 1949. James Watt and the history of steam power. New York: Henry Schuman, Inc.
Pritchard, P. 2011. Fox and McDonald's introduction to fluid mechanics, 8th ed. New York: Wiley.

Blair Stringam is a College Associate Professor in the Department of Plant and Environmental Sciences at NMSU. He earned a Ph.D. in agricultural and biological engineering at Utah State University. His work focuses on irrigation, water management, water measurement, and rainwater harvesting.
To find more resources for your business, home, or family, visit the College of Agricultural, Consumer and Environmental Sciences on the World Wide Web at pubs.nmsu.edu.
Contents of publications may be freely reproduced for educational purposes. All other rights reserved. For permission to use publications for other purposes, contact pubs@nmsu.edu or the authors listed on the publication.
New Mexico State University is an equal opportunity/affirmative action employer and educator. NMSU and the U.S. Department of Agriculture cooperating.
Printed and electronically published August 2013 Las Cruces, NM